Trigonometry
Worked Examples
The Unit Circle
We discussed trigonometric values of angles in a right-angle triangle, namely angles less than $90^{\circ}$ or $\pi/2$ rad. What about greater angles?Consider the unit circle in the coordinate plane centered at the origin. Let $A$ be a point on the circle in the first quadrant and $\theta$ denote the angle it makes with the $x$-axis. Then the coordinates of $A$ are exactly $\left(x,y\right)=\left(\cos\left(\theta\right),\sin\left(\theta\right)\right)$. (Details)To see this, consider the perpendicular from $A$ to the $x$-axis, denote the endpoint $H$. The triangle $AHO$ is a right-angle triangle with side lengths of $OH$, $AH$, and $OA$ being $x$, $y$ and $1$ respectively. Then $\sin\left(\theta\right)=y/1=y$, $\cos\left(\theta\right)=x/1=x$.
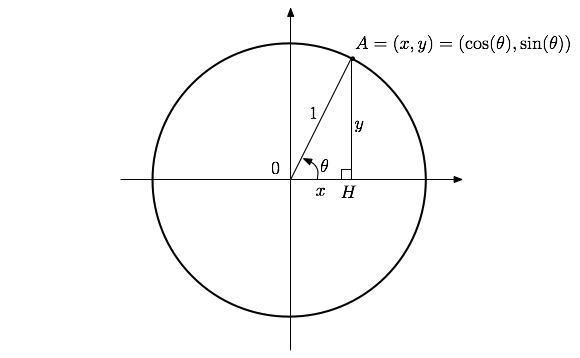
We can extend this to any angle $\theta$: Think of the hands of a clock, one being fixed at the $x$-axis and the other going around counterclockwise in the unit circle to make an angle $\theta$ with the first one. The point the second hand reaches on the unit circle has coordinates $\left(\cos\left(\theta\right),\sin\left(\theta\right)\right)$.
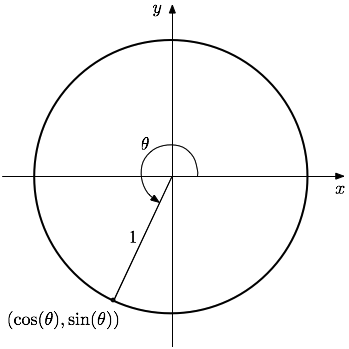
Note: Going around the circle once measures an angle of $2 \pi$ rad. To get larger angles, we go around the circle more than once, say for $5 \pi/2$ we go around once and keep going until we reach the $y$-axis.
To get negative angles, we go clockwise, say for $-\pi/2$, starting from the $x$-axis we go clockwise until reaching the $y$-axis.
Example. Find the trigonometric values of $\frac{\pi}{2}$.
At $\frac{\pi}{2}$, the hand of the clock is pointing at $\left(0,1\right)$. So, $\cos\left(\frac{\pi}{2}\right)=0, \sin\left(\frac{\pi}{2}\right)=1, \cot\left(\frac{\pi}{2}\right)=0/1=0$ and $\tan$ is not defined at $\frac{\pi}{2}$. A nice way of remembering which trigonometric functions take a positive or a negative value in each quadrant is the CAST rule: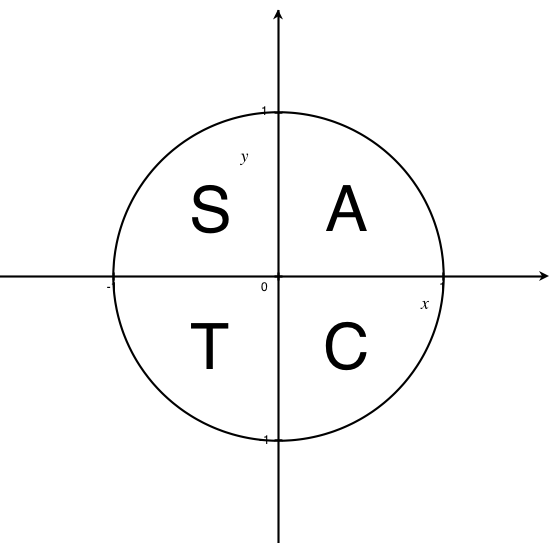
| C | $\cos(\theta)$ is positive there |
| A | all trigonometric functions are positive there |
| S | $\sin(\theta)$ is positive there |
| T | $\tan(\theta)$ is positive there |
Some of the more important angles along with the corresponding coordinates are illustrated below.

