Trigonometry

Radians

So far, we have been using degrees to measure angles. Another unit of measurement is radians.

One radian is the angle which creates an arc the same lenth as $r$, the radius of the circle.



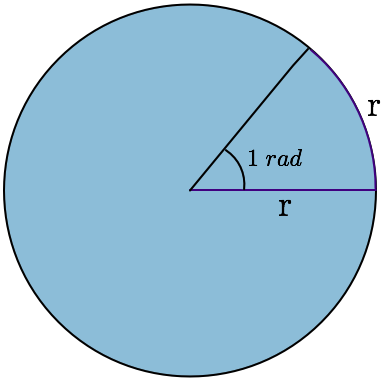
How do we go between degrees and radians?

Recall that the angle created when we go around the circle is $360^{\circ}$. The arc created is the circumference, i.e. $2\pi r$, so the measurement of that angle is also $2\pi$ radians. So, we get: $$360^{\circ}=2\pi \hspace{3 mm} \text{radians}$$ This tells us that $1^{\circ}=2\pi/360 \hspace{3 mm} \text{rad}$, or: $$\theta^{\circ}=\frac{2\pi}{360}\times \theta \hspace{3 mm} \text{rad}$$ And similarly: $$\theta\hspace{3 mm} \text{rad}=\frac{360}{2 \pi}\times \theta^{\circ}$$

Example. Compute $45^{\circ}$ in radians and $7 \hspace{3 mm} \text{rad}$ in degrees.

$$45^{\circ}=\frac{2\left(45\right)\pi}{360}\hspace{3 mm} \text{rad}=\frac{\pi}{4} \hspace{3 mm} \text{rad}$$ $$7 \hspace{3 mm} \text{rad}=\frac{360\left(7\right)}{2 \pi}^{\circ}=\frac{1260}{\pi}^{\circ}\approx 401^{\circ}$$